November 6, 2024
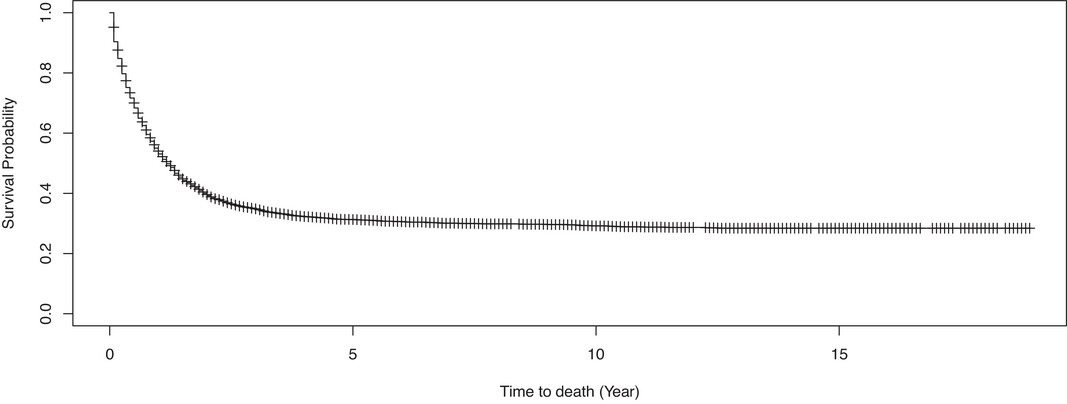
The literature has been lacking in ways to model sufficient follow-up when analyzing survival data with a cure fraction, which means that the right extreme of the censoring time distribution is larger than that of the survival distribution of the susceptibles. Some researchers came up with some solutions for this but these do not work well when there is not enough information in the right tail of the survival function. The authors have developed a novel testing procedure from which they modified the extreme value distribution and their test statistic tests the difference between the cured rate and the non-cured rate so they can check for sufficient follow-up. They used a bootstrap procedure to obtain critical values and p-values for their test statistic. They established the asymptotic normality of their test statistic. Their proposed test was based on the condition that the F0 distribution for the non-cured population is the Gumbel domain of attraction. Since there are no known tests to estimate this Gumbel domain then they suggested estimating the extreme value index and rejecting the Gumbel condition of the estimate exceeds a given threshold.
The authors conducted simulations and a real dataset analysis, from the SEER cancer database. Their test statistic performed well. They haven’t yet extended this method to include covariates but are planning to do so.
Written by,
Usha Govindarajulu, PhD
Keywords: survival, cure fraction, susceptibles, Gumbel, extreme value
References
Xie P, Escobar-Bach M, and Keilegom IV (2024) “Testing for Sufficient Follow-Up in Censored Survival Data by Using Extremes”. Biometrical Journal. https://onlinelibrary.wiley.com/doi/10.1002/bimj.202400033
https://onlinelibrary.wiley.com/cms/asset/e9762a74-1c00-4f56-9e66-70a15a4b88f7/bimj2622-fig-0001-m.jpg